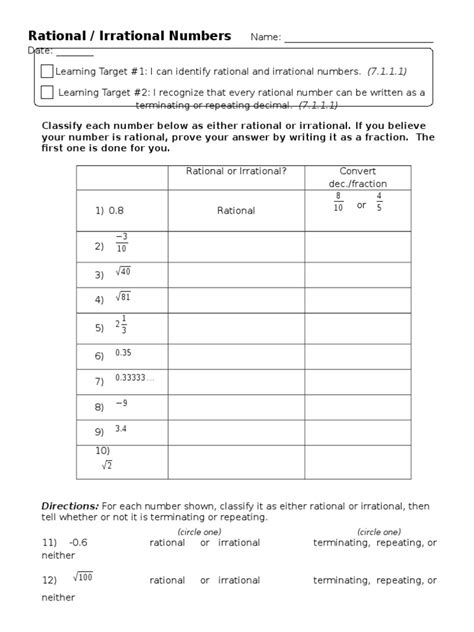
This worksheet is designed to help you understand the concepts of rational and irrational numbers. It will provide you with examples and explanations of the different types of numbers and how they relate to one another. It will also explore the different ways that they can be used in everyday life. By the end of this worksheet, you should have a better understanding of rational and irrational numbers, and how to use them in your own life.

Exploring the Relationship Between Rational and Irrational Numbers with a Worksheet

Exploring the Relationship Between Rational and Irrational Numbers

The mathematics of rational and irrational numbers is an important concept to understand. Rational numbers are those that can be expressed as the ratio of two integers, while irrational numbers are those that cannot be expressed as the ratio of two integers. In this worksheet, we will explore the relationship between these two types of numbers and discuss how they can be used in everyday life.

Part 1: Defining Rational and Irrational Numbers

Rational numbers are numbers that can be expressed as the ratio of two integers, such as 1/2, 3/4, or 9/10. They can also be written as a decimal, such as 0.5, 0.75, and 0.9. Rational numbers can be represented on a number line, and they can also be expressed as a fraction.

Irrational numbers are numbers that cannot be expressed as the ratio of two integers. Examples of irrational numbers include the square root of a number (√2, √3, etc.), the square root of a negative number (√-1, √-2, etc.), and pi (π). Irrational numbers cannot be represented on a number line, and they cannot be expressed as a fraction.

Part 2: Examples of Rational and Irrational Numbers

Rational numbers: 1/2, 3/4, 9/10, 0.5, 0.75, 0.9

Irrational numbers: √2, √3, √-1, √-2, π

Part 3: Using Rational and Irrational Numbers in Everyday Life

Rational and irrational numbers can be used in everyday life in a variety of ways. For example, in cooking and baking, fractions can be used to measure ingredients, and irrational numbers such as pi can be used to calculate the circumference of a circle. In construction, fractions can be used to measure distances, and irrational numbers such as the square root of two can be used to calculate the length of a diagonal line.

Part 4: Summary

Rational and irrational numbers are two types of numbers that have distinct properties and uses. Rational numbers are numbers that can be expressed as the ratio of two integers, and they can be represented on a number line and expressed as a fraction. Irrational numbers are numbers that cannot be expressed as the ratio of two integers, and they cannot be represented on a number line or expressed as a fraction. Both types of numbers can be used in everyday life, such as in cooking, baking, and construction.

How to Identify Rational and Irrational Numbers with a Worksheet

A rational number is any number that can be expressed as a fraction, with a numerator (top number) and a denominator (bottom number). An irrational number is any number that cannot be expressed as a fraction, meaning it is not a ratio of two integers. Identifying rational and irrational numbers can be a challenging task, but with practice it can become easier.

This worksheet is designed to help you practice identifying rational and irrational numbers. For each type of number, you will be provided with a list of numbers and asked to indicate which ones are rational and which ones are irrational. After completing the worksheet, you should have a better understanding of how to determine if a number is rational or irrational.

Instructions: For each set of numbers, indicate whether the number is rational or irrational.

1. 8 _____ | 3.2 _____

2. 𝜋 _____ | 4.7 _____

Answer: 1. Rational | 3.2 Rational
2. Irrational | 4.7 Rational

Applying the Properties of Rational and Irrational Numbers with a Worksheet

Rational and irrational numbers are two key concepts in mathematics. It is important for students to understand the properties of each type of number and how to differentiate between them. To help students gain a better understanding of rational and irrational numbers, this worksheet offers a range of activities that apply the properties of each type of number.

Activity 1: Identifying Rational Numbers

Instructions: Read the following numbers and decide if they are rational or irrational.

1. pi
Answer: Irrational

2. 0.25
Answer: Rational

3. -3
Answer: Rational

4. √2
Answer: Irrational

Activity 2: Comparing Rational and Irrational Numbers

Instructions: Compare the following pairs of numbers, and explain why they are different.

1. pi and 0.25
Answer: Pi is an irrational number and 0.25 is a rational number. This is because pi is an infinite, non-repeating decimal, whereas 0.25 is a fraction that can be represented as a terminating decimal.

2. -3 and √2
Answer: -3 is a rational number and √2 is an irrational number. This is because -3 can be expressed as a fraction, whereas √2 is an infinite, non-repeating decimal.

Analyzing Real-Life Examples of Rational and Irrational Numbers with a Worksheet

Introduction

The concept of rational and irrational numbers can be somewhat confusing to many students. In this worksheet, we will explore the differences between rational and irrational numbers by looking at several real-life examples. By the end of this worksheet, you should be able to distinguish between rational and irrational numbers and understand why some numbers are classified as one or the other. Let’s get started!

Rational Numbers

Rational numbers are numbers that can be written as a ratio of two integers. Examples of rational numbers include fractions, integers, and repeating decimals.

1. What is the rational number equivalent of the fraction 8/15?

The rational number equivalent of the fraction 8/15 is 0.53.

2. What is the rational number equivalent of the repeating decimal 0.3333…?

The rational number equivalent of the repeating decimal 0.3333… is 1/3.

Irrational Numbers

Irrational numbers are numbers that cannot be written as a ratio of two integers. Examples of irrational numbers include square roots and pi.

3. What is the irrational number equivalent of the square root of 25?

The irrational number equivalent of the square root of 25 is 5.

4. What is the irrational number equivalent of pi?

The irrational number equivalent of pi is 3.14159265358979323846264338327950288419716939937510582097494459230781640628620899862803482534211706798.

Conclusion

By analyzing these real-life examples of rational and irrational numbers, you should now have a better understanding of the differences between the two. Rational numbers can be written as a ratio of two integers, while irrational numbers cannot be written as a ratio of two integers. With this knowledge, you should now be able to quickly tell whether a number is rational or irrational. Good luck!

Practicing Problem-Solving with Rational and Irrational Numbers Using a Worksheet

Problem-solving with rational and irrational numbers can be a challenging task for students. To help them practice this skill, a worksheet can be a great way to practice and learn. This worksheet will help students work through problems involving rational and irrational numbers.

First, students should identify the number as either rational or irrational. Rational numbers are numbers that can be expressed as a fraction, while irrational numbers cannot be expressed as a fraction.

Next, students should practice solving equations with rational and irrational numbers. This can involve solving equations with both types of numbers, or solving equations with only one type. Students should work through each equation to determine the outcome.

Then, students should practice solving inequalities with rational and irrational numbers. This involves solving equations with both types of numbers, or solving equations with only one type. They should work through each inequality to determine the outcome.

Finally, students should practice solving problems with both types of numbers. This involves using both rational and irrational numbers in the same problem. Students should work through each problem to determine the outcome.

By working through the problems on this worksheet, students will gain practice in identifying and solving problems with rational and irrational numbers. They will also gain an understanding of how to use both types of numbers to solve problems.

Images of Rational And Irrational Numbers Worksheet

Download Rational And Irrational Numbers Worksheet

Download Rational And Irrational Numbers Worksheet: Click Here

Conclusion

The Rational And Irrational Numbers Worksheet is a great tool to help students understand the concepts of rational and irrational numbers. Through this worksheet, students can gain an understanding of how to differentiate between the two different types of numbers, as well as how to identify and classify them. This worksheet can be used to reinforce existing knowledge and to help guide students through the process of identifying rational and irrational numbers. With the help of this worksheet, students can become better equipped to recognize and classify these numbers in the future.

Some pictures about 'Rational And Irrational Numbers Worksheet'

Related posts of "Rational And Irrational Numbers Worksheet"