Dimensional Analysis Practice Worksheet

Dimensional analysis: what is it?

It helps to have a basic understanding of units and dimensions in order to tackle mathematical issues involving physical quantities. The fundamental idea behind dimensions is that only quantities that share the same dimension can be added or removed. We can determine relationships between physical quantities thanks to this idea.

The study of the relationship between physical quantities based on their dimensions and unit systems is known as dimensional analysis. It is applied when converting a unit between two different forms. The units must remain constant when answering mathematical problems in order to make the task simple.

Understand the importance of dimensional analysis? Well! Dimensional analysis is a technique used in engineering and science to explain the relationships between various physical quantities based on their fundamental characteristics, such as length, mass, time, and electric current, as well as units of measurement like miles vs. kilometres, or pounds vs. kilograms.

In other words, we study both fundamental and derived physical quantities in physics. The seven fundamental units are time, luminous intensity, mass, length, amount of substance, and electric current. However, derived amounts result when we combine two or more fundamental units.

We use the symbols M for mass, L for length, and so forth. Similar to distance/time, we use M/L to express speed, which is a derived number.

or [ML-1]. We arrive at the dimensional formula of multiple values in this way.

Based on the unit we want in the answer, a conversion factor is utilized. We will also calculate the dimensional formulas for the different values on this page.

How Should Dimensional Analysis Be Done?

Conversion of units

Since a conversion factor is employed to evaluate the units, dimensional analysis is also known as a unit factor method or factor label method.

As an illustration, let’s say we want to know how many meters there are in 4 kilometers.

In most cases, we calculate as

A km is equal to a m.

1 kilometre divided by 4 equals 4000 meters.

(1000 meters is used as the conversion factor here.)

The Homogeneity of Dimensional Analysis Principle

According to this principle, “all equations that represent physical units have the same dimensions. An equation cannot accurately represent a physical situation if two of its sides don’t share the same dimensions.

An illustration of dimension analysis

The values must correspond to the same quantity in order to use a conversion factor. For instance, 60 minutes equals 1 hour, 1000 meters equals 1 kilometer, and 12 months equals 1 year.

Let’s attempt to comprehend things in this manner. Consider having 15 pens. If you multiply that amount by 1, you will still have 15 pens. You need the conversion factor to determine how many packets of the pen are equivalent to 15 pens.

Image of Dimensional Analysis Practice Worksheet

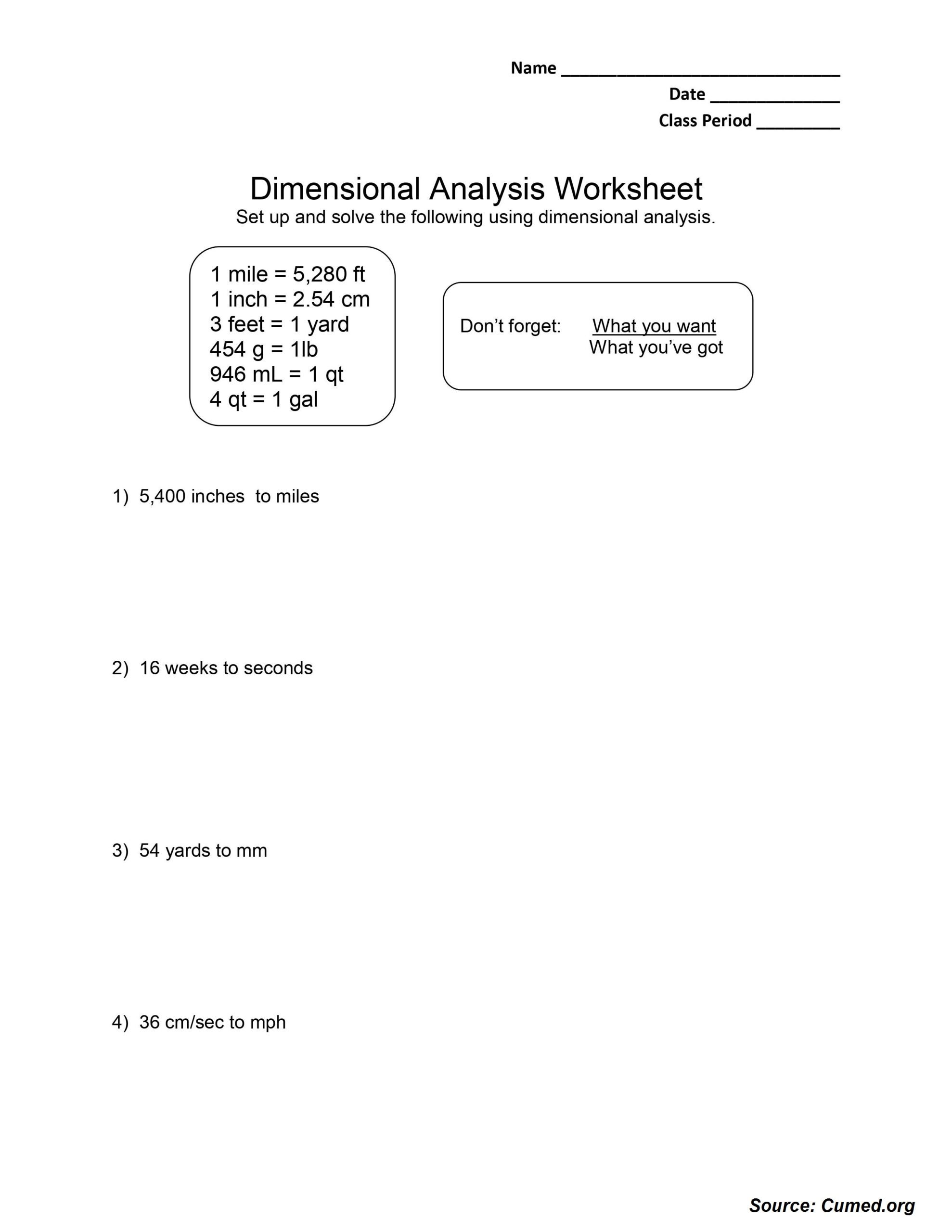
Download Dimensional Analysis Practice Worksheet PDF

Download Dimensional Analysis Practice Worksheet PDF: Click Here

Uses for Dimensional Analysis

Dimensional analysis is a crucial component of measurement and is used extensively in physics. The five following arguments are the key justifications for using dimensional analysis:

• to confirm the accuracy of an equation or any other physical relationship based on the homogeneity principle. Dimensions must exist on both sides of the equation. If the LHS and RHS of an equation have the same dimensions, the dimensional relation will be accurate. The relations will also be inaccurate if the dimensions on two sides are off.
• The value of a physical quantity can be changed from one system of units to another by using dimensional analysis.
• It is a symbol for the character of a physical amount.
• Dimensional expressions can be changed using algebraic quantities.
• The creation of formulas involves dimensional analysis.

Dimensional analysis’s limitations

1. Dimensional analysis has certain significant drawbacks, including:
2. The dimensional constant is not disclosed via dimensional analysis.
3. Exponential, logarithmic, and trigonometric functions cannot be derived by dimensional analysis.
4. It does not reveal whether a physical quantity is a scalar or a vector.

Derivation for Kinetic Energy, an illustration of a dimensional formula

The mathematical statement that expresses the powers to which the fundamental units (mass M, length L, and time T) are to be increased in order to obtain one unit of a derived quantity is known as a physical entity’s dimensional formula.

Let’s use an example to further grasp the dimensional formula now. Knowing that kinetic energy is one of the essential components of physics, its formula is crucial in many areas of the study of physics. So let’s calculate the kinetic energy in dimensions formula.

Related posts of "Dimensional Analysis Practice Worksheet"